MINLPLib
A Library of Mixed-Integer and Continuous Nonlinear Programming Instances
Home // Instances // Documentation // Download // Statistics
Instance bayes2_30
| Formatsⓘ | ams gms lp mod nl osil pip py |
| Primal Bounds (infeas ≤ 1e-08)ⓘ | |
| Other points (infeas > 1e-08)ⓘ | |
| Dual Boundsⓘ | 0.00000000 (ANTIGONE) 0.00010741 (BARON) 0.00000000 (COUENNE) 0.00010509 (GUROBI) 0.00000000 (LINDO) 0.00000000 (SCIP) 0.00000000 (SHOT) |
| Referencesⓘ | Greenberg, Betsy, Lasdon, L S, and Plummer, John, Using Global Optimization to Estimate Population Class Sizes, Journal of Global Optimization, 36:3, 2006, 319-338. |
| Sourceⓘ | Leon Lasdon |
| Added to libraryⓘ | 31 Jul 2001 |
| Problem typeⓘ | QCP |
| #Variablesⓘ | 86 |
| #Binary Variablesⓘ | 0 |
| #Integer Variablesⓘ | 0 |
| #Nonlinear Variablesⓘ | 65 |
| #Nonlinear Binary Variablesⓘ | 0 |
| #Nonlinear Integer Variablesⓘ | 0 |
| Objective Senseⓘ | min |
| Objective typeⓘ | linear |
| Objective curvatureⓘ | linear |
| #Nonzeros in Objectiveⓘ | 20 |
| #Nonlinear Nonzeros in Objectiveⓘ | 0 |
| #Constraintsⓘ | 77 |
| #Linear Constraintsⓘ | 22 |
| #Quadratic Constraintsⓘ | 55 |
| #Polynomial Constraintsⓘ | 0 |
| #Signomial Constraintsⓘ | 0 |
| #General Nonlinear Constraintsⓘ | 0 |
| Operands in Gen. Nonlin. Functionsⓘ | |
| Constraints curvatureⓘ | indefinite |
| #Nonzeros in Jacobianⓘ | 598 |
| #Nonlinear Nonzeros in Jacobianⓘ | 440 |
| #Nonzeros in (Upper-Left) Hessian of Lagrangianⓘ | 770 |
| #Nonzeros in Diagonal of Hessian of Lagrangianⓘ | 0 |
| #Blocks in Hessian of Lagrangianⓘ | 1 |
| Minimal blocksize in Hessian of Lagrangianⓘ | 65 |
| Maximal blocksize in Hessian of Lagrangianⓘ | 65 |
| Average blocksize in Hessian of Lagrangianⓘ | 65.0 |
| #Semicontinuitiesⓘ | 0 |
| #Nonlinear Semicontinuitiesⓘ | 0 |
| #SOS type 1ⓘ | 0 |
| #SOS type 2ⓘ | 0 |
| Minimal coefficientⓘ | 5.8431e-06 |
| Maximal coefficientⓘ | 4.2400e+02 |
| Infeasibility of initial pointⓘ | 9945 |
| Sparsity Jacobianⓘ | 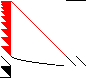 |
| Sparsity Hessian of Lagrangianⓘ |  |
$offlisting
*
* Equation counts
* Total E G L N X C B
* 78 68 10 0 0 0 0 0
*
* Variable counts
* x b i s1s s2s sc si
* Total cont binary integer sos1 sos2 scont sint
* 87 87 0 0 0 0 0 0
* FX 0
*
* Nonzero counts
* Total const NL DLL
* 619 179 440 0
*
* Solve m using NLP minimizing objvar;
Variables x1,x2,x3,x4,x5,x6,x7,x8,x9,x10,x11,x12,x13,x14,x15,x16,x17,x18,x19
,x20,x21,x22,x23,x24,x25,x26,x27,x28,x29,x30,x31,x32,x33,x34,x35,x36
,x37,x38,x39,x40,x41,x42,x43,x44,x45,x46,x47,x48,x49,x50,x51,x52,x53
,x54,x55,x56,x57,x58,x59,x60,x61,x62,x63,x64,x65,x66,x67,x68,x69,x70
,x71,x72,x73,x74,x75,x76,x77,x78,x79,x80,x81,x82,x83,x84,x85,x86
,objvar;
Positive Variables x1,x2,x3,x4,x5,x6,x7,x8,x9,x10,x11,x12,x13,x14,x15,x16,x17
,x18,x19,x20,x21,x22,x23,x24,x25,x26,x27,x28,x29,x30,x31,x32,x33,x34
,x35,x36,x37,x38,x39,x40,x41,x42,x43,x44,x45,x46,x47,x48,x49,x50,x51
,x52,x53,x54,x55,x56,x57,x58,x59,x60,x61,x62,x63,x64,x65,x66,x67,x68
,x69,x70,x71,x72,x73,x74,x75,x76,x77,x78,x79,x80,x81,x82,x83,x84,x85
,x86;
Equations e1,e2,e3,e4,e5,e6,e7,e8,e9,e10,e11,e12,e13,e14,e15,e16,e17,e18,e19
,e20,e21,e22,e23,e24,e25,e26,e27,e28,e29,e30,e31,e32,e33,e34,e35,e36
,e37,e38,e39,e40,e41,e42,e43,e44,e45,e46,e47,e48,e49,e50,e51,e52,e53
,e54,e55,e56,e57,e58,e59,e60,e61,e62,e63,e64,e65,e66,e67,e68,e69,e70
,e71,e72,e73,e74,e75,e76,e77,e78;
e1.. 0.299999999999999*x2 - x12*(0.299999999999999*x2 + 0.420042004200419*x3 +
0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e2.. 0.420042004200419*x3 - x13*(0.299999999999999*x2 + 0.420042004200419*x3 +
0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e3.. 0.0899789978997899*x3 - x14*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e4.. 0.441069311972204*x4 - x15*(0.299999999999999*x2 + 0.420042004200419*x3 +
0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e5.. 0.188993694328424*x4 - x16*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e6.. 0.026981099790315*x4 - x17*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11) =E= 0
;
e7.. 0.411670573863023*x5 - x18*(0.299999999999999*x2 + 0.420042004200419*x3 +
0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e8.. 0.264632763149874*x5 - x19*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e9.. 0.0755697503379837*x5 - x20*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11) =E= 0
;
e10.. 0.00808866220581916*x5 - x21*(0.00808866220581916*x5 + 0.0283216463789187
*x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*
x9 + 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e11.. 0.360201456247447*x6 - x22*(0.299999999999999*x2 + 0.420042004200419*x3
+ 0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e12.. 0.308773522162667*x6 - x23*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e13.. 0.132281083087121*x6 - x24*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e14.. 0.0283216463789187*x6 - x25*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e15.. 0.00242433293003544*x6 - x26*(0.00242433293003544*x6 + 0.0101872919521249
*x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e16.. 0.30254760034903*x7 - x27*(0.299999999999999*x2 + 0.420042004200419*x3 +
0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e17.. 0.324235367869763*x7 - x28*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e18.. 0.185233220498984*x7 - x29*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e19.. 0.0594967092564436*x7 - x30*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e20.. 0.0101872919521249*x7 - x31*(0.00242433293003544*x6 + 0.0101872919521249*
x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e21.. 0.000726450938014618*x7 - x32*(0.000726450938014618*x7 +
0.00356174664425821*x8 + 0.0099784508208397*x9 + 0.0209655320789341*x10
+ 0.0367069932830306*x11) =E= 0;
e22.. 0.247052285556172*x8 - x33*(0.299999999999999*x2 + 0.420042004200419*x3
+ 0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e23.. 0.317759744553093*x8 - x34*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e24.. 0.22694961744096*x8 - x35*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e25.. 0.0972085184322643*x8 - x36*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e26.. 0.0249702818996627*x8 - x37*(0.00242433293003544*x6 + 0.0101872919521249*
x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e27.. 0.00356174664425821*x8 - x38*(0.000726450938014618*x7 +
0.00356174664425821*x8 + 0.0099784508208397*x9 + 0.0209655320789341*x10
+ 0.0367069932830306*x11) =E= 0;
e28.. 0.000217629988834878*x8 - x39*(0.000217629988834878*x8 +
0.00121958164462655*x9 + 0.00384420873582218*x10 + 0.00897533537960286*
x11) =E= 0;
e29.. 0.197610748687493*x9 - x40*(0.299999999999999*x2 + 0.420042004200419*x3
+ 0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e30.. 0.296571521818452*x9 - x41*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e31.. 0.25421627517133*x9 - x42*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e32.. 0.136128890917752*x9 - x43*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e33.. 0.0466305167574201*x9 - x44*(0.00242433293003544*x6 + 0.0101872919521249*
x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e34.. 0.0099784508208397*x9 - x45*(0.000726450938014618*x7 +
0.00356174664425821*x8 + 0.0099784508208397*x9 + 0.0209655320789341*x10
+ 0.0367069932830306*x11) =E= 0;
e35.. 0.00121958164462655*x9 - x46*(0.000217629988834878*x8 +
0.00121958164462655*x9 + 0.00384420873582218*x10 + 0.00897533537960286*
x11) =E= 0;
e36.. 6.51822832565586e-5*x9 - x47*(6.51822832565586e-5*x9 +
0.000410977166249314*x10 + 0.00143951015242667*x11) =E= 0;
e37.. 0.15558731597959*x10 - x48*(0.299999999999999*x2 + 0.420042004200419*x3
+ 0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e38.. 0.266899105175344*x10 - x49*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e39.. 0.266949986712887*x10 - x50*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e40.. 0.171561639066158*x10 - x51*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e41.. 0.0734703645857939*x10 - x52*(0.00242433293003544*x6 + 0.0101872919521249
*x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e42.. 0.0209655320789341*x10 - x53*(0.000726450938014618*x7 +
0.00356174664425821*x8 + 0.0099784508208397*x9 + 0.0209655320789341*x10
+ 0.0367069932830306*x11) =E= 0;
e43.. 0.00384420873582218*x10 - x54*(0.000217629988834878*x8 +
0.00121958164462655*x9 + 0.00384420873582218*x10 + 0.00897533537960286*
x11) =E= 0;
e44.. 0.000410977166249314*x10 - x55*(6.51822832565586e-5*x9 +
0.000410977166249314*x10 + 0.00143951015242667*x11) =E= 0;
e45.. 1.95181536733007e-5*x10 - x56*(1.95181536733007e-5*x10 +
0.000136750150848869*x11) =E= 0;
e46.. 0.120982941684104*x11 - x57*(0.299999999999999*x2 + 0.420042004200419*x3
+ 0.441069311972204*x4 + 0.411670573863023*x5 + 0.360201456247447*x6 +
0.30254760034903*x7 + 0.247052285556172*x8 + 0.197610748687493*x9 +
0.15558731597959*x10 + 0.120982941684104*x11) =E= 0;
e47.. 0.233513342319487*x11 - x58*(0.0899789978997899*x3 + 0.188993694328424*x4
+ 0.264632763149874*x5 + 0.308773522162667*x6 + 0.324235367869763*x7 +
0.317759744553093*x8 + 0.296571521818452*x9 + 0.266899105175344*x10 +
0.233513342319487*x11) =E= 0;
e48.. 0.266961437732522*x11 - x59*(0.026981099790315*x4 + 0.0755697503379837*x5
+ 0.132281083087121*x6 + 0.185233220498984*x7 + 0.22694961744096*x8 +
0.25421627517133*x9 + 0.266949986712887*x10 + 0.266961437732522*x11)
=E= 0;
e49.. 0.200192450750305*x11 - x60*(0.00808866220581916*x5 + 0.0283216463789187*
x6 + 0.0594967092564436*x7 + 0.0972085184322643*x8 + 0.136128890917752*x9
+ 0.171561639066158*x10 + 0.200192450750305*x11) =E= 0;
e50.. 0.102892337231952*x11 - x61*(0.00242433293003544*x6 + 0.0101872919521249*
x7 + 0.0249702818996627*x8 + 0.0466305167574201*x9 + 0.0734703645857939*
x10 + 0.102892337231952*x11) =E= 0;
e51.. 0.0367069932830306*x11 - x62*(0.000726450938014618*x7 +
0.00356174664425821*x8 + 0.0099784508208397*x9 + 0.0209655320789341*x10
+ 0.0367069932830306*x11) =E= 0;
e52.. 0.00897533537960286*x11 - x63*(0.000217629988834878*x8 +
0.00121958164462655*x9 + 0.00384420873582218*x10 + 0.00897533537960286*
x11) =E= 0;
e53.. 0.00143951015242667*x11 - x64*(6.51822832565586e-5*x9 +
0.000410977166249314*x10 + 0.00143951015242667*x11) =E= 0;
e54.. 0.000136750150848869*x11 - x65*(1.95181536733007e-5*x10 +
0.000136750150848869*x11) =E= 0;
e55.. 5.84313858841381e-6*x11 - 5.84313858841381e-6*x66*x11 =E= 0;
e56.. 0.300000000000001*x2 - 424*x12 - x67 + x77 =E= 0;
e57.. 0.510021002100211*x3 - 424*x13 - 208*x14 - x68 + x78 =E= 0;
e58.. 0.657044106090946*x4 - 424*x15 - 208*x16 - 243*x17 - x69 + x79 =E= 0;
e59.. 0.7599617495567*x5 - 424*x18 - 208*x19 - 243*x20 - 191*x21 - x70 + x80
=E= 0;
e60.. 0.83200204080619*x6 - 424*x22 - 208*x23 - 243*x24 - 191*x25 - 79*x26
- x71 + x81 =E= 0;
e61.. 0.882426640864363*x7 - 424*x27 - 208*x28 - 243*x29 - 191*x30 - 79*x31
- 31*x32 - x72 + x82 =E= 0;
e62.. 0.917719824515245*x8 - 424*x33 - 208*x34 - 243*x35 - 191*x36 - 79*x37
- 31*x38 - 10*x39 - x73 + x83 =E= 0;
e63.. 0.942421168101182*x9 - 424*x40 - 208*x41 - 243*x42 - 191*x43 - 79*x44
- 31*x45 - 10*x46 - 2*x47 - x74 + x84 =E= 0;
e64.. 0.959708647654471*x10 - 424*x48 - 208*x49 - 243*x50 - 191*x51 - 79*x52
- 31*x53 - 10*x54 - 2*x55 - x75 + x85 =E= 0;
e65.. 0.971806941822881*x11 - 424*x57 - 208*x58 - 243*x59 - 191*x60 - 79*x61
- 31*x62 - 10*x63 - 2*x64 - x76 + x86 =E= 0;
e66.. x2 + 2*x3 + 3*x4 + 4*x5 + 5*x6 + 6*x7 + 7*x8 + 8*x9 + 9*x10 + 10*x11
=E= 10000;
e67.. x2 + x3 + x4 + x5 + x6 + x7 + x8 + x9 + x10 + x11 =G= 424;
e68.. x3 + x4 + x5 + x6 + x7 + x8 + x9 + x10 + x11 =G= 208;
e69.. x4 + x5 + x6 + x7 + x8 + x9 + x10 + x11 =G= 243;
e70.. x5 + x6 + x7 + x8 + x9 + x10 + x11 =G= 191;
e71.. x6 + x7 + x8 + x9 + x10 + x11 =G= 79;
e72.. x7 + x8 + x9 + x10 + x11 =G= 31;
e73.. x8 + x9 + x10 + x11 =G= 10;
e74.. x9 + x10 + x11 =G= 2;
e75.. x10 + x11 =G= 0;
e76.. x11 =G= 0;
e77.. - x1 + x2 + x3 + x4 + x5 + x6 + x7 + x8 + x9 + x10 + x11 =E= 0;
e78.. - x67 - x68 - x69 - x70 - x71 - x72 - x73 - x74 - x75 - x76 - x77 - x78
- x79 - x80 - x81 - x82 - x83 - x84 - x85 - x86 + objvar =E= 0;
* set non-default bounds
x2.up = 10000;
x3.up = 10000;
x4.up = 10000;
x5.up = 10000;
x6.up = 10000;
x7.up = 10000;
x8.up = 10000;
x9.up = 10000;
x10.up = 10000;
x11.up = 10000;
x12.up = 1;
x13.up = 1;
x14.up = 1;
x15.up = 1;
x16.up = 1;
x17.up = 1;
x18.up = 1;
x19.up = 1;
x20.up = 1;
x21.up = 1;
x22.up = 1;
x23.up = 1;
x24.up = 1;
x25.up = 1;
x26.up = 1;
x27.up = 1;
x28.up = 1;
x29.up = 1;
x30.up = 1;
x31.up = 1;
x32.up = 1;
x33.up = 1;
x34.up = 1;
x35.up = 1;
x36.up = 1;
x37.up = 1;
x38.up = 1;
x39.up = 1;
x40.up = 1;
x41.up = 1;
x42.up = 1;
x43.up = 1;
x44.up = 1;
x45.up = 1;
x46.up = 1;
x47.up = 1;
x48.up = 1;
x49.up = 1;
x50.up = 1;
x51.up = 1;
x52.up = 1;
x53.up = 1;
x54.up = 1;
x55.up = 1;
x56.up = 1;
x57.up = 1;
x58.up = 1;
x59.up = 1;
x60.up = 1;
x61.up = 1;
x62.up = 1;
x63.up = 1;
x64.up = 1;
x65.up = 1;
x66.up = 1;
x67.up = 1000;
x68.up = 1000;
x69.up = 1000;
x70.up = 1000;
x71.up = 1000;
x72.up = 1000;
x73.up = 1000;
x74.up = 1000;
x75.up = 1000;
x76.up = 1000;
x77.up = 1000;
x78.up = 1000;
x79.up = 1000;
x80.up = 1000;
x81.up = 1000;
x82.up = 1000;
x83.up = 1000;
x84.up = 1000;
x85.up = 1000;
x86.up = 1000;
* set non-default levels
x2.l = 1;
x3.l = 1;
x4.l = 1;
x5.l = 1;
x6.l = 1;
x7.l = 1;
x8.l = 1;
x9.l = 1;
x10.l = 1;
x11.l = 1;
x12.l = 0.01;
x13.l = 0.01;
x14.l = 0.01;
x15.l = 0.01;
x16.l = 0.01;
x17.l = 0.01;
x18.l = 0.01;
x19.l = 0.01;
x20.l = 0.01;
x21.l = 0.01;
x22.l = 0.01;
x23.l = 0.01;
x24.l = 0.01;
x25.l = 0.01;
x26.l = 0.01;
x27.l = 0.01;
x28.l = 0.01;
x29.l = 0.01;
x30.l = 0.01;
x31.l = 0.01;
x32.l = 0.01;
x33.l = 0.01;
x34.l = 0.01;
x35.l = 0.01;
x36.l = 0.01;
x37.l = 0.01;
x38.l = 0.01;
x39.l = 0.01;
x40.l = 0.01;
x41.l = 0.01;
x42.l = 0.01;
x43.l = 0.01;
x44.l = 0.01;
x45.l = 0.01;
x46.l = 0.01;
x47.l = 0.01;
x48.l = 0.01;
x49.l = 0.01;
x50.l = 0.01;
x51.l = 0.01;
x52.l = 0.01;
x53.l = 0.01;
x54.l = 0.01;
x55.l = 0.01;
x56.l = 0.01;
x57.l = 0.01;
x58.l = 0.01;
x59.l = 0.01;
x60.l = 0.01;
x61.l = 0.01;
x62.l = 0.01;
x63.l = 0.01;
x64.l = 0.01;
x65.l = 0.01;
x66.l = 0.01;
Model m / all /;
m.limrow=0; m.limcol=0;
m.tolproj=0.0;
$if NOT '%gams.u1%' == '' $include '%gams.u1%'
$if not set NLP $set NLP NLP
Solve m using %NLP% minimizing objvar;
Last updated: 2025-08-07 Git hash: e62cedfc